Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Lexikon:

Länge von Kurven

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Übersicht

Berechne jeweils die Länge der Kurve $ \gamma$ .

  1. $ \gamma:[0,1]\to\mathbb{R}^3$ , $ \gamma(t)=(t,t^2,\frac{2}{3}\;t^3)^\mathrm{t}$ .
  2. $ \gamma:[0,2\pi]\to\mathbb{R}^2$ , $ \gamma(t)=(t-\sin t,1-\cos t)^\mathrm{t}$ (Zykloide).
  3. $ \gamma:[0,2\pi n]\to\mathbb{R}^3$ , $ \gamma(t)=(r\cos t,r\sin t,ht)^\mathrm{t}$ , wobei $ n\ge 1$ und $ h,\, r\in\mathbb{R}_{> 0}$ (Schraubenlinie mit $ n$ Umdrehungen, Radius $ r$ und Steigung $ h$ ).

Lösung.

  1. Es wird

    $\displaystyle \ell(\gamma) \;=\; \int_0^1 \left\Vert\dot{\gamma}(t)\right\Vert\... ...2)\;\mathrm{d}t \;=\; \left[t+\frac{2}{3}\;t^3\right]_0^1 \;=\; \frac{5}{3}\;. $

    Skizze.

    \includegraphics[width = 8cm]{l1-1.eps}

  2. Es wird

    \begin{displaymath} \begin{array}{rcl} \ell(\gamma) &=& \displaystyle\int_0^{2\p... ...frac{t}{2}\right]_0^{2\pi}\vspace*{2mm}\\ &=& 8\;. \end{array}\end{displaymath}

    Dabei haben wir folgende Identität verwendet.

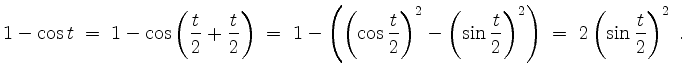
    $\displaystyle 1-\cos t \;=\; 1-\cos\left(\frac{t}{2}+\frac{t}{2}\right) \;=\; 1... ...\left(\sin\frac{t}{2}\right)^2\right) \;=\; 2\left(\sin\frac{t}{2}\right)^2\;. $

    Skizze.

    \includegraphics[width = 8cm]{l1-2.eps}

  3. Es wird

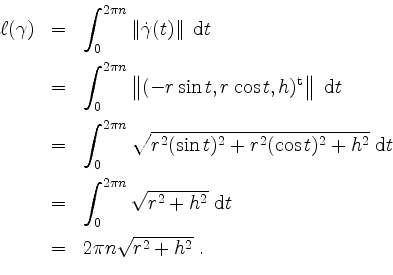
    \begin{displaymath} \begin{array}{rcl} \ell(\gamma) &=& \displaystyle\int_0^{2\p... ...hrm{d}t\vspace*{2mm}\\ &=& 2\pi n\sqrt{r^2+h^2}\;. \end{array}\end{displaymath}

    Skizze für $ n=2$ , $ r=1$ und $ h=1$ .

    \includegraphics[width = 8cm]{l1-3.eps}

(Autoren: Künzer/Martin/Tentler/Wahrheit)

siehe auch:

  automatisch erstellt am 11.  8. 2006