Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Lexikon:

Flächenberechnung mit dem Gauß'schen Integralsatz

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Übersicht
Es soll der Flächeninhalt des Gebiets $ A$, das von einer Ellipse $ C$ mit Halbachsenlängen $ a, b > 0$ berandet wird, berechnet werden.

Parametrisiert man die Randkurve $ C$ durch

\begin{displaymath} \vec{r}(t)=\left( \begin{array}{c} a \cos t\\ b \sin t\\ \end{array}\right),\quad t\in [0,2\pi]\,, \end{displaymath}

so erhält man für den Flächeninhalt mit dem Satz von Gauß
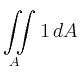$\displaystyle \iint\limits_A 1 \,dA$ $\displaystyle =$ \begin{displaymath}\frac{1}{2} \int\limits_{C} \vec{r} \times d \vec{r} = \frac{... ...n{array}{r} -a \sin t\,dt\\ b \cos t\,dt\\ \end{array}\right)\end{displaymath}  
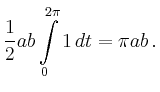  $\displaystyle =$ $\displaystyle \frac{1}{2} ab \int\limits_0^{2\pi} 1 \,dt =\pi ab\,.$  

[Verweise]
  automatisch erstellt am 9. 10. 2013