Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Lexikon:

Beispiel: Univariate Fourier-Transformation

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Übersicht
Gesucht wird die Fourier-Transformation der Funktion

$\displaystyle f(x)=e^{-\vert x\vert}\,. $

Mit der Formel von Euler-Moivre folgt e$ ^{-\text{i}xy} =\cos (xy)-\text{i}\sin(xy)$. Da $ f$ gerade ist, ist $ \int_{-\infty}^\infty f(x) \sin(xy) dx =0 $ und

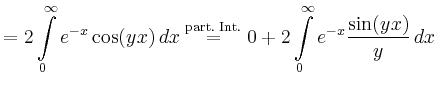$\displaystyle \hat{f}(y)$ $\displaystyle = 2\int\limits_0^\infty e^{-x}\cos(yx)\,dx \overset{\text{part. Int.}}{=} 0+2\int\limits_0^\infty e^{-x}\frac{\sin(yx)}{y}\,dx$    
  $\displaystyle \overset{\text{part. Int.}}{=} 2\left[e^{-x}\left(-\frac{\cos(yx)... ...}\right)\right]_0^\infty - 2\int\limits_0^\infty e^{-x}\frac{\cos(yx)}{y^2}\,dx$    
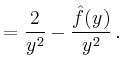  $\displaystyle = \frac{2}{y^2} - \frac{\hat{f}(y)}{y^2}\,.$    

Aufgelöst ergibt sich


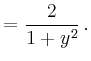$\displaystyle \hat{f}(y)$ $\displaystyle = \frac{2}{1+y^2}\,.$    

siehe auch:

  automatisch erstellt am 13. 11. 2013