Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen] Englische Flagge
Mathematik-Online-Lexikon:

Partielle Integration von Wurzelausdrücken

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z Übersicht
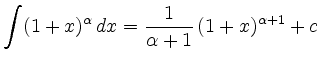Aus $ {\displaystyle{\int (1+x)^\alpha\, dx=\frac{1}{\alpha+1}\,(1+x)^{\alpha+1}+c}}$ für $ \alpha\neq -1$ folgt
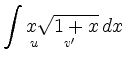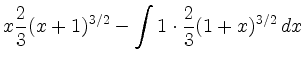$\displaystyle \int \underset{u \quad\:\: v'\quad}{x\sqrt{1+x}}\,dx$ $\displaystyle =$ $\displaystyle x\frac{2}{3}(x+1)^{3/2} - \int 1\cdot\frac{2}{3}(1+x)^{3/2}\,dx$  
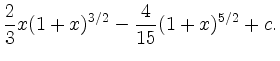  $\displaystyle =$ $\displaystyle \frac{2}{3}x(1+x)^{3/2} - \frac{4}{15}(1+x)^{5/2} + c.$  

Analog berechnet man
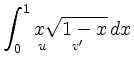$\displaystyle \int_0^1 \underset{u \quad \:\: v'\quad}{x\sqrt{1-x}}\,dx$ $\displaystyle =$ $\displaystyle \left[ -x\frac{2}{3}(1-x)^{3/2} \right]_0^1 + \int_0^1 1\cdot\frac{2}{3}(1-x)^{3/2}\,dx$  
  $\displaystyle =$ $\displaystyle 0 - \left[ \frac{4}{15} (1-x)^{5/2} \right]_0^1 = \frac{4}{15}\,.$  

(Autoren: Höllig/Kopf)

siehe auch:

  automatisch erstellt am 8.  4. 2008