Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Aufgabensammlung: Lösung zu

Aufgabe 1400: Integralberechnung durch Substitution

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
Berechnen Sie durch geeignete Substitution die folgenden Integrale.
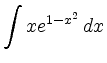
a) $ \displaystyle\int x e^{1-x^2}\, d x$                 b) $ \displaystyle\int \cos(x)e^{\sin(x)}\, dx$
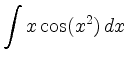
c) $ \displaystyle\int \frac{\big(\ln(x)\big)^n}{x}\, d x$   mit $ n\in\mathbb{N}$                 d) $ \displaystyle\int x\cos(x^2)\, d x$
Für geeignete Intervalle $ I_1, I_2\subseteq \mathbb{R}$ und eine geeignete Substitutionsfunktion $ u\colon I_1\rightarrow I_2\colon x\mapsto u(x)$ gilt bekanntlich bei der Integration der Funktion $ f\colon I_2\rightarrow\mathbb{R}$ mit der zugehörigen Stammfunktion $ F$:

$\displaystyle \int f(u)\, d u=\int f(u(x))\,u'(x)\, d x=\big[F\circ u\big] $

a)
Für $ f_1\colon u\mapsto e^u$ und die Substitution $ u_1\colon x\mapsto 1-x^2$ erhält man $ u_1'(x)=-2x$ und damit

$\displaystyle \int x e^{1-x^2}\, d x= -\frac{1}{2}\int e^{u_1} \, d u_1=-\frac{... ...(u)\, d x \left[-\frac{1}{2}e^{u_1}\right]=\left[-\frac{1}{2}e^{1-x^2}\right]\,$.

b)
Für $ f_2\colon u\mapsto e^u$ und die Substitution $ u_2\colon x\mapsto \sin(x)$ erhält man $ u_2'(x)=\cos(x)$ und damit

$\displaystyle \int \cos(x)e^{\sin(x)}\, d x =\int e^{u_2}\, d u_2=\left[e^{u_2}\right]=\left[e^{\sin(x)}\right]\,$.

c)
Für $ f_3\colon u\mapsto u^n$ und die Substitution $ u_3\colon x\mapsto \ln(x)$ erhält man $ u_3'(x)=\frac{1}{x}$ und damit

$\displaystyle \int \frac{\big(\ln(x)\big)^n}{x}\, d x =\int u_3^n\, d u_3=\left[\frac{1}{n+1}u_3^{n+1}\right] =\left[\frac{1}{n+1}\big(\ln(x)\big)^{n+1}\right]\,$.

d)
Für $ f_4\colon u\mapsto \cos(u)$ und die Substitution $ u_4\colon x\mapsto x^2$ erhält man $ u_4'(x)=2x$ und damit

$\displaystyle \int x\cos(x^2)\, d x =\frac{1}{2} \int \cos(u_4)\, d u_4= \left[\frac{1}{2} \sin(u_4)\right]= \left[\frac{1}{2} \sin(x^2)\right]\,$.

(Ackermann/Poppitz)
[Zurück zur Aufgabe]
  automatisch erstellt am 25.  8. 2006