Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Aufgabensammlung: Lösung zu

Aufgabe 1338: Kurvenintegral

A B C D E F G H I J K L M N O P Q R S T U V W X Y Z
Berechnen Sie für die Funktion

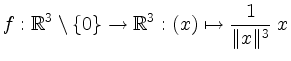
$\displaystyle f:\mathbb{R}^3\setminus\{0\}\to\mathbb{R}^3: (x)\mapsto\frac{1}{\Vert x\Vert^3}\;x $

das Kurvenintegral über das sogenannte Vivianische Fenster

$\displaystyle \gamma(t)=\left( \begin{array}{c} \cos t\\ \sin t \\ 2\sin \frac{t}{2} \end{array}\right), \quad 0 \leqslant t\leqslant 4\pi. $

Wir geben drei Lösungsmöglichkeiten an.

  1. Wir wollen mit dem zweiten Hauptsatz für Kurvenintegrale beweisen, daß $ f$ konservativ ist. Es gilt

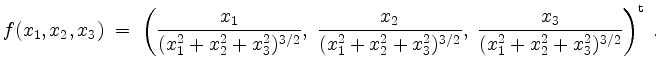
    $\displaystyle f(x_1,x_2,x_3) \;=\; \left(\frac{x_1}{(x_1^2+x_2^2+x_3^2)^{3/2}},... ...^2+x_3^2)^{3/2}},\; \frac{x_3}{(x_1^2+x_2^2+x_3^2)^{3/2}}\right)^\mathrm{t}\;. $

    Also ist

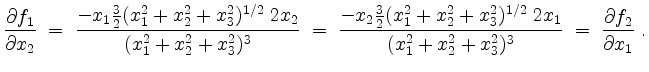
    $\displaystyle \dfrac{\partial f_1}{\partial x_2} \;=\; \dfrac{-x_1\frac{3}{2}(x... ...1/2}\;2x_1}{(x_1^2+x_2^2+x_3^2)^3} \;=\; \dfrac{\partial f_2}{\partial x_1}\;. $

    Aus Symmetriegründen ist daher auch

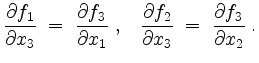
    $\displaystyle \dfrac{\partial f_1}{\partial x_3} \;=\; \dfrac{\partial f_3}{\pa... ...\dfrac{\partial f_2}{\partial x_3} \;=\; \dfrac{\partial f_3}{\partial x_2}\;. $

    Also erfüllt $ f$ die Integrabilitätsbedingungen. Da das Gebiet $ \mathbb{R}^3\setminus\{0\}$ einfach zusammenhängend ist, ist das Vektorfeld $ f$ nach dem zweiten Hauptsatz für Kurvenintegral konservativ. Da der Weg $ \gamma$ geschlossen ist, folgt also

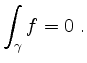
    $\displaystyle \int_\gamma f=0\;. $

  2. Wir berechnen direkt eine Stammfunktion von $ f$ . Es gilt

    $\displaystyle \Vert x\Vert' \;=\; \frac{1}{\Vert x\Vert}\;x $

    für $ x\in\mathbb{R}^n\setminus\{0\}$ , wie man direkt verifiziert. Ist $ \alpha\ne 0$ , so folgt mit der Kettenregel

    $\displaystyle \left(\Vert x\Vert^\alpha\right)' \;=\; \alpha\Vert x\Vert^{\alpha-1}\cdot (\Vert x\Vert)' \;=\; \alpha\Vert x\Vert^{\alpha-2}\;x\;. $

    Speziell gilt also mit $ \alpha=-1$

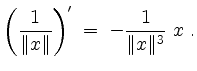
    $\displaystyle \left(\frac{1}{\Vert x\Vert}\right)' \;=\; -\frac{1}{\Vert x\Vert^3}\;x\;. $

    Also ist die Funktion

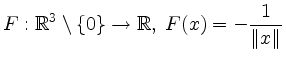
    $\displaystyle F:\mathbb{R}^3\setminus\{0\}\to\mathbb{R},\; F(x)=-\frac{1}{\Vert x\Vert} $

    eine Stammfunktion von $ f$ . Mithin ist das Vektorfeld $ f$ konservativ, und es folgt $ \int_\gamma f=0$ , da der Weg $ \gamma$ geschlossen ist.

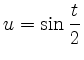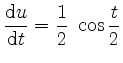
  3. Wir berechnen $ \int_\gamma f$ direkt mit der Definition. Es wird mit der Substitution $ u=\sin\dfrac{t}{2}$ und $ \dfrac{\mathrm{d}u}{\mathrm{d}t}=\dfrac{1}{2}\;\cos\dfrac{t}{2}$

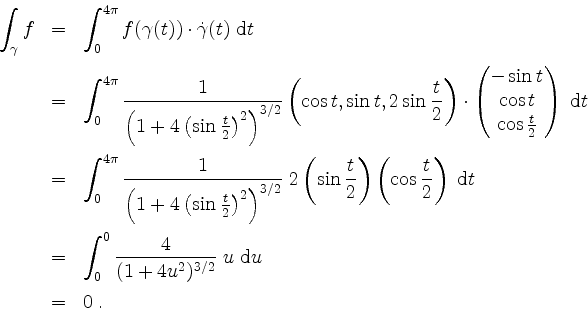
    \begin{displaymath} \begin{array}{rcl} \displaystyle\int_\gamma f &=& \displayst... ...2)^{3/2}}\; u\;\mathrm{d}u\vspace*{2mm}\\ &=& 0\;. \end{array}\end{displaymath}

Skizze des Vivianischen Fensters.

\includegraphics[width = 8cm]{s2.eps}
(Autoren: Künzer/Martin/Tentler/Wahrheit)
[Zurück zur Aufgabe]
  automatisch erstellt am 11.  8. 2006