
Mathematik-Online-Kurs: Formelsammlung - Fourier-Analysis

|
[Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen] |
|
Mathematik-Online-Kurs: Formelsammlung - Fourier-Analysis | |
Multivariate Fourier-Transformation |
| [vorangehende Seite] | [Gesamtverzeichnis][Seitenübersicht] |
| Fourier-Transformation |
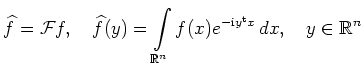 |
|
| Inverse Fourier-Transformation |
 |
|
Transformationsregeln
| Linearität |
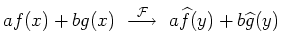 |
|||||||||
| Symmetrie |
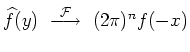 |
|||||||||
| Transformation |
 |
|||||||||
| Verschiebung |
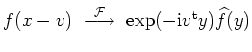 |
|||||||||
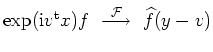 |
||||||||||
| Differentiation |
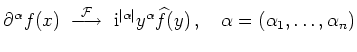 |
|||||||||
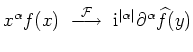 |
||||||||||
| Faltung |
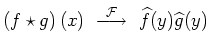 |
|||||||||
| Spezielle Funktionen | ||||||||||
|
||||||||||
| Norminvarianz |
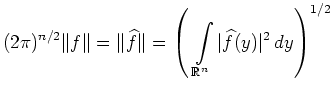 |
|||||||||
| [vorangehende Seite] | [Gesamtverzeichnis][Seitenübersicht] |
| automatisch erstellt am 31.1.2006 |