Mo Logo [Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen]
Mathematik-Online-Kurs: Gruppentheorie - Klassische Matrixgruppen - Lorentz-Gruppe

Struktur der eigentlichen orthochronen Gruppe

[vorangehende Seite] [nachfolgende Seite] [Gesamtverzeichnis][Seitenübersicht]
Wir betrachten noch mal die Gruppe $ SO^{+}(3,1)$. Diese Gruppe enthält folgende spezielle Elemente:
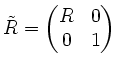  1. Lorentz-Drehungen: $ \tilde R = \begin{pmatrix}R & 0\\ 0 & 1\end{pmatrix}$ mit $ R \in SO(3)$.
  2. Lorentz-Boosts: $ B = \begin{pmatrix}1 & 0 & 0 & 0\\ 0 & 1 & 0 & 0\\ 0 & 0 & cosh\,t& sinh\,t\\ 0 & 0 & sinh\,t & cosh\,t\end{pmatrix}$, $ \hspace{0.3cm} t \in \mathbb{R}$.
Zu jedem $ A \in SO^{+}(3,1)$ gibt es Matrizen $ R,S \in SO(3)$ und $ t \in \mathbb{R}$, so dass

$\displaystyle A = \tilde{R} \cdot B(t) \cdot \tilde{S}.$

Insbesondere wird $ SO^{+}(3,1)$ von den Lorentz-Drehungen und den Lorentz-Boosts erzeugt.

(Autor: Borgart)
Sei $ A = \begin{pmatrix}C & x \\ y^{t} & a \end{pmatrix} \in SO^{+}(3,1)$ mit $ a \in \mathbb{R}, \hspace{0.2cm} a \geq 1.$ Dann gilt wegen $ A \in O(3,1)$:

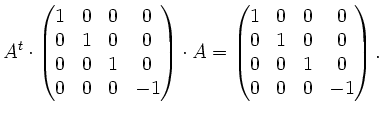
$\displaystyle A^{t} \cdot \begin{pmatrix}1 & 0 & 0 & 0\\ 0 & 1 & 0 & 0\\ 0 & 0 ... ...ix}1 & 0 & 0 & 0\\ 0 & 1 & 0 & 0\\ 0 & 0 & 1& 0\\ 0 & 0 & 0 & -1\end{pmatrix}.$

Durch Ausmultiplizieren erhält man die dazu äquivalenten Gleichungen

$\displaystyle C^{t}C = E_{3} + yy^{t}$ (1)

$\displaystyle x^{t}x +1 = a^{2}$ (2)

$\displaystyle C^{t}x = ay$ (3)

Wegen $ a \geq 1$ gibt es ein $ t \in \mathbb{R}$ so dass $ cosh \, t = a.$ Mit (2) folgt daraus $ \hspace{0.15cm}\vert x\vert = \vert(0, 0, sinh \,t)^t\vert.$
Also gibt es eine Matrix $ R \in SO(3)$ so dass

$\displaystyle R^{-1}x = \begin{pmatrix}0 \\ 0 \\ sinh \, t \end{pmatrix}.$

Nun setzen wir $ T = B(-t)\cdot \tilde{R} \cdot A$. Dann gilt $ T \in SO(3,1)$ und $ T \cdot e_{4} = e_{4}.$ Damit gilt: $ T = \begin{pmatrix}S & 0 \\ y^{t} & 1 \end{pmatrix}.$
Wegen (3) ist $ y = (0,0,0)^{t}$ und mit (1) folgt daraus, dass

$\displaystyle S^{t} \cdot S = E_{3}, \hspace{0.2cm} \rm {d. h.} \hspace{0.15cm} S \in SO(3).$

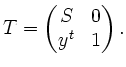
Also ist $ T = \begin{pmatrix}S & 0 \\ 0 & 1 \end{pmatrix}, \hspace{0.15cm} S \in SO(3)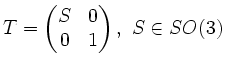$ und damit folgt insgesamt

$\displaystyle A= \tilde{R} \cdot B(-t)^{-1} \cdot \tilde{S} = \tilde{R} \cdot B(t) \cdot \tilde{S}.\\ \\ $

(Autor: Borgart)
[vorangehende Seite] [nachfolgende Seite] [Gesamtverzeichnis][Seitenübersicht]
  automatisch erstellt am 14.11.2008